Решить систему методом постановки
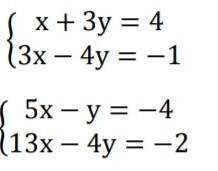
Другие вопросы по теме Алгебра
Популярные вопросы
- Вычислить производную y=x^(arctg(x^(1/...
1 - Какова выделительная система ракообращных...
3 - Деталь объемом 0.004м( в кубе) имеет массу 86кг определите плотность вещества...
3 - Придумайте страшилку ( без аморальных вещей )...
2 - Найдите значение выражение 38a+62 при a=238; 489...
1 - Какую массу соли можно получить из 10 кг морской воды содержание солей в которой...
3 - Написать 15 пар слов с беглыми гласными...
3 - Подготовить mini-dialoge о том, куда бы вы хотели поехать и чем там делать на...
1 - Мама развела 27 л яблочного сока в трехлитровой банке и 4 л в двухлитровый сколько...
3 - Определите массу воды, которую надо добавить к 20 г раствора серной кислоты с...
1
Объяснение: