Знайдіть область визначення функції 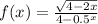
Другие вопросы по теме Алгебра
Популярные вопросы
- Write the missing letters...
1 - Вычислите отношения веоичин 300кг/6т...
3 - Эссе как жили древние люди...
3 - Как работает кожух индукционной тигельной печи? (желательно подробно)...
3 - У прямокутному тр-ку ABC( кут C= 90 градусов) проведена бисектрыса BD...
3 - Для каких алкенов в ряду характерна геометрическая изомерия? пентен-2,бутен-1,4-метил-3-гептен,2-бутен...
3 - Опис осіннього дня з образами: нюховий, слуховий,зоровий,смаковий тактильний....
2 - тока под цифрой нужно с лева начертить рисунок) а справа дано т.д. снизу...
2 - 9. Вал вращается с частотой п=180об/мин. С некоторого момента вал начинает...
2 - Что изучают вс исторические дисциплины истории:АРХЕОЛОГИЯ, ЭТГОГРАФИЯ,...
2
Объяснение: