 найти:
найти: 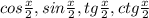
Другие вопросы по теме Алгебра
Популярные вопросы
- Решить примеры. 1)40+(x+16)=93 2)49(x+160)=102 3)58+(x+16)=111...
3 - При взаимодействии 12г магния с 196г серной кислоты выделяется водород объёмом...
3 - Ураганный ветер движется со скоростью 108 км/ч.за какое время он пройдет расстояние...
2 - Столы в первом классе имеют высоту 52 см . во втором классе споры на 2 см. выше...
1 - Заполни пропуски. 68 мм + ? мм = 1 дм, 96 м + ? м =1 км, 29 см +? см =1м, 8 мм...
2 - 3k+0,5(1-6k)-7-6 при к= 0,05 , к=1,2, к=10 полный ответ...
3 - Обж 7 класс вопрос почему возникают сели,оползни,обвалы,лавины...
3 - 1ч 26мин+2ч34мин 2ч30мин--55мин 13т250кг+820кг...
1 - Read and fill in may or may not. пример 1)a. may i see jackson,please? b.certainly....
3 - Чем похожи сказки пушкина.а.с (сказка о царе салтане) и жуковсковоюа.в( сказка...
1
tgx/2;cosx/2;sinx/2;ctgx/2=??
1)1+tg²x=1/cos²x
cos²x=1/(1+tg²x)=1/(1+16/9)=9/25
cosx=+√9/25=3/5
2)3π/2<x<2π
3π/4<x/2<π
3)sin²x/2=(1-cosx)/2=(1-3/5)/2=2/5*1/2=1/5
sinx/2=+√(1/5)=√5/5
4)cos²x/2=(1+cosx)/2=(1+3/5)/2=8/5*1/2=4/5
cosx/2=-√(4/5)=-2√5/5
5)tg²x/2=(1-cosx)/(1+cosx)=(1-3/5)/(1+3/5)=
2/5/8/5=2/5*5/8=1/4
tgx/2=-√(1/4)=-1/2
6)ctg²x/2=(1+cosx)/(1-cosx)=
(1+3/5)/(1-3/5)=8/5*5/2=4
ctgx/2=-√4=-2