Решите уравнение 1) 2cos^2x+cosx-1=0 2)4sin^2+11sinx-3=0 3)v3 tgx - v3 ctgx=2 4) sin2x+v3 cos2x=1
Другие вопросы по теме Алгебра
Популярные вопросы
- Кожей каких рыб традиционно покрывают рукояти самурайских мечей?...
2 - решить какие слитно а какие раздельно...
3 - Просмотрев предложенные видео в хоu Tube дo Exзaню ответ вопросы:1....
1 - с физикой, нужны все 4 задачи День рождения 21 число. Заранее...
2 - Медленно тратим потому что ничего не знаем)...
3 - ТЕКСТ: Сколько бы ни смотреть на море – оно никогда не надоест....
3 - Физика тжб 2 токсан Комектесындерш...
1 - Суйдынбайдын акындык бейнесын корсететын олен жолын аныкта...
2 - 1) Що таке програмне забезпечення комп’ютера? * 1.Сукупність периферійних...
3 - Программа 7 класса, хотя-бы половинчатые ответы ПРОИЗВОДСТВЕННАЯ...
2
1)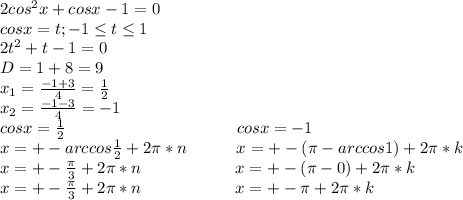
n и k принадлежат Z.
2.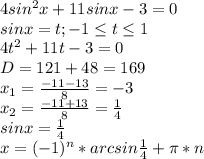
n принадлежит Z. -3 исключаем т.к. неуд. условию.
3.
n и k принадлежат Z.
4.Напишу долгий и нудный. 2рой лёгкий(введение вс угла)
1)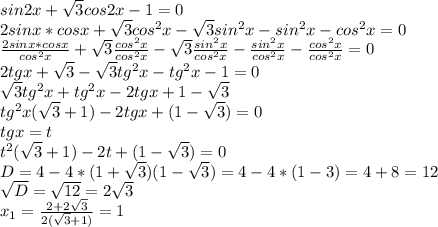
2)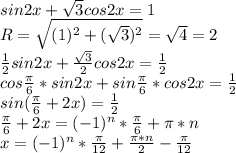
Зря наверно писал))
Во вложении
----------------------------------------------