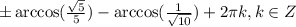решить:
Корень(3)*sin(x)-cos(x)=-корень(2)
Другие вопросы по теме Математика
Популярные вопросы
- Open the brackets and make the story complete one day a paris newspaper...
2 - Дано: угол аб доказать: угол аб меньше или равен 180°...
2 - Які тварини з довгими до 7 см серповитними пазурами вилазять на...
2 - Запишите уравнение реакции сероводорода с раствором нитрата свинца(ii)...
1 - Объясните пословицы: 1) прямым путем по кривой не ездят. 2) всё...
2 - Может ли одно и тоже животное обитать разных средах примеры...
1 - Из 60 вопросов на экзамене, студент выучил 40. какая вероятность...
1 - Написать тему и идею к сказке о мертвой царевне и семи богатырях...
2 - Указать первообразную функцию f(x)=2x+4x в кубе-1...
1 - Предумайте на нахождение кокого либо придмета...
1
Пусть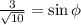 . Тогда
. Тогда 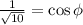
С учетом того, что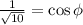 ,
, 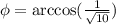
Окончательно имеем: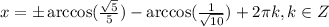
ОТВЕТ: